How can the value of current through an inductance be determined at very low frequencies?
How to Determine the Current through an Inductor at Extremely Low Frequencies
When operating at extremely low frequencies (such as DC or near-DC frequencies), the current flowing through an inductor can be determined by analyzing the behavior of the circuit. Since an inductor exhibits very low impedance at DC or extremely low frequencies, it can almost be considered a short circuit. However, for more precise determination of the current at these frequencies, several factors need to be considered:
1. DC Resistance (DCR) of the Inductor
An inductor is not an ideal component; it has a certain amount of wire resistance known as the DC resistance (DCR). At extremely low frequencies or DC conditions, the inductive reactance (XL=2πfL) is negligible, so the current is primarily limited by the inductor's DC resistance.
If the circuit consists only of an inductor and a power source, with the inductor's DC resistance being RDC , the current I can be calculated using Ohm's Law:
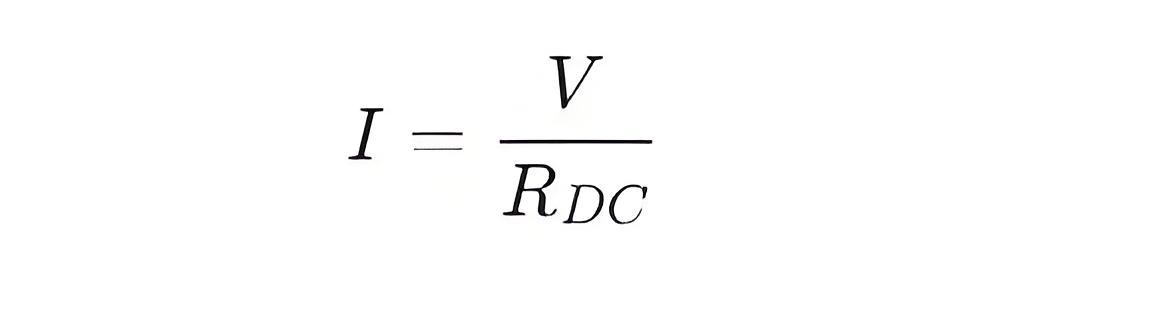
where V is the supply voltage.
2. Effect of the Time Constant
At extremely low frequencies, the current through the inductor does not immediately reach its steady-state value but gradually increases to that value. This process is governed by the circuit's time constant τ, which is defined as:
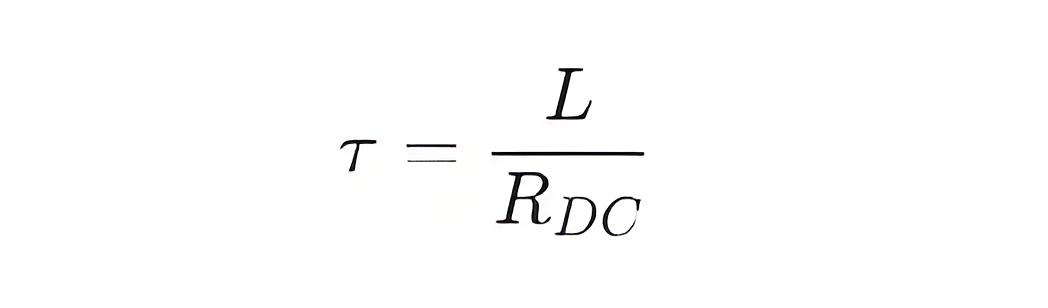
where L is the inductance and R DC is the inductor's DC resistance.The current as a function of time can be described by the following equation
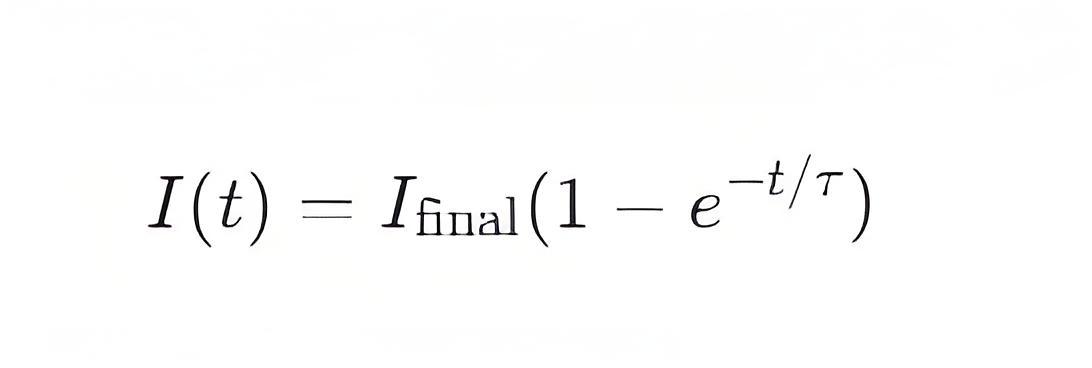
where Ifinal =V/RDC is the steady-state current, and t is time.
This means that the current starts at zero and gradually increases, reaching approximately 99% of its steady-state value after about 5τ.
3. Type of Power Source
DC Power Source: If the power source is a constant DC voltage, the current will eventually stabilize at I=V/R DC after sufficient time.
Extremely Low Frequency AC Power Source: If the power source is a sinusoidal or pulsed waveform at an extremely low frequency, the current will vary with the instantaneous voltage of the source. For an extremely low-frequency sine wave, the peak current can be approximated as:
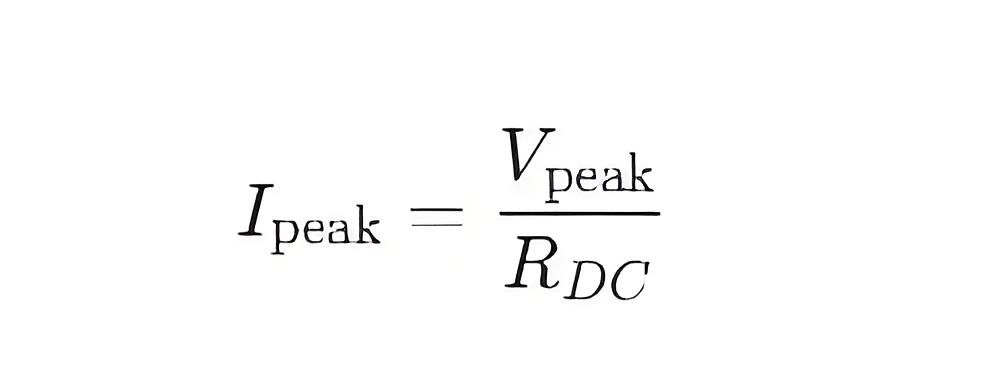
where V peak is the peak voltage of the source.
4. Other Components in the Circuit
If the circuit contains other components besides the inductor (such as resistors or capacitors), their effects on the current must be considered. For example, in an RL circuit, the rate at which the current grows is influenced by both the resistance R and the inductance L, with the time constant τ=L/R.
If the circuit includes a capacitor, the charging and discharging of the capacitor will also affect the current, especially during transient periods.
5. Non-Ideal Effects of the Inductor
Real inductors may have parasitic capacitance and core losses. At extremely low frequencies, the effect of parasitic capacitance is usually negligible, but core losses can cause the inductor to heat up, affecting its performance. If the inductor uses magnetic material (such as an iron core), magnetic saturation can also be an issue, especially under high current conditions. When an inductor saturates, its inductance L drops significantly, leading to a rapid increase in current.
6. Measurement Methods
Steady-State Current Measurement: To measure the steady-state current, a current meter can be used to directly measure the current flowing through the inductor once the circuit has reached a stable state.
Transient Current Measurement: To measure the current as it changes over time, an oscilloscope or another instrument capable of capturing transient responses can be used. By observing the current waveform, you can analyze how the current grows and reaches its final value.
7. Special Case: Magnetic Saturation
If the inductor uses magnetic material (such as an iron core), it may enter a state of magnetic saturation at high currents or strong magnetic fields. When the inductor saturates, its inductance L decreases significantly, causing the current to rise rapidly. To avoid magnetic saturation, ensure that the operating current does not exceed the inductor's maximum rated current.
Summary
At extremely low frequencies, the current through an inductor is primarily determined by the inductor's DC resistance RDC , and the current growth is controlled by the time constant τ=L/RDC . For a DC power source, the current will eventually stabilize at I=V/RDC . For an extremely low-frequency AC power source, the instantaneous current depends on the instantaneous voltage of the source. Additionally, the presence of other circuit components and non-ideal characteristics of the inductor (such as magnetic saturation) should be considered.
The Electricity Encyclopedia is dedicated to accelerating the dissemination and application of electricity knowledge and adding impetus to the development and innovation of the electricity industry.