How do I calculate the fault current at the secondary side of a transformer which is supplying a transmission line with a certain impedance?
Calculating the fault current (short-circuit current) on the secondary side of a transformer supplying a transmission line is a complex process that involves multiple parameters of the power system. Below are the steps and relevant formulas to help you understand how to perform this calculation. We will assume the system is a three-phase AC system, and the fault occurs on the transformer's secondary side.
1. Determine System Parameters
Transformer Parameters:
Rated capacity of the transformer rated S rated (unit: MVA)
Transformer impedance ZT (usually given as a percentage, e.g., ZT =6%)
Primary-side voltage of the transformer V1(unit: kV)
Secondary-side voltage of the transformer V2 (unit: kV)
Transmission Line Parameters:
Impedance of the transmission line ZL (unit: ohms or ohms per kilometer)
Length of the transmission line L (unit: kilometers)
Equivalent Source Impedance:
The equivalent impedance of the source ZS (unit: ohms), typically provided by the upstream grid. If the source is very strong (e.g., from a large power plant or infinite bus), you can assume ZS ≈0.
2. Normalize All Impedances to the Same Base
To simplify calculations, it's common to normalize all impedances to the same base value (usually the primary or secondary side of the transformer). Here, we choose to normalize all impedances to the secondary side of the transformer.
Base Voltage: Select the secondary-side voltage V2 as the base voltage.
Base Capacity: Choose the rated capacity of the transformer rated Srated as the base capacity.
The base impedance is calculated as:
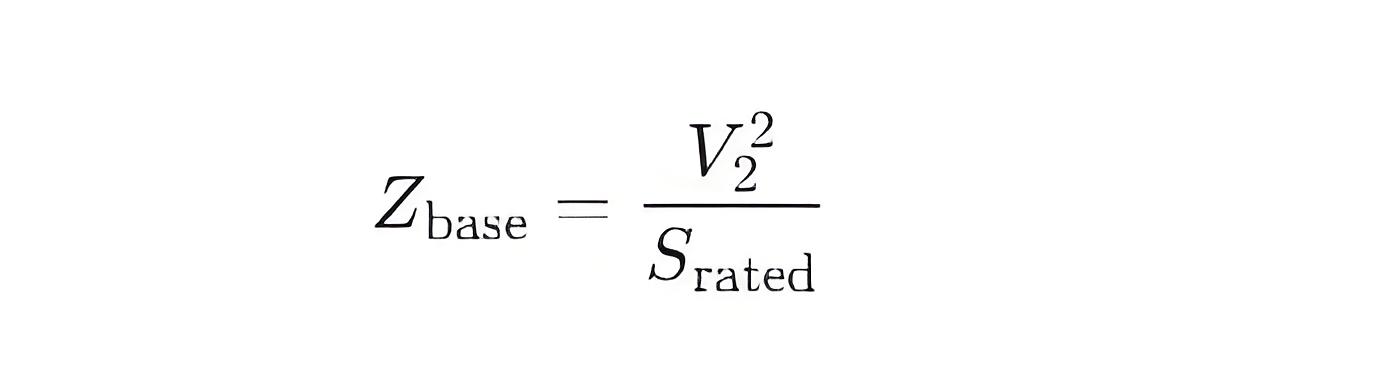
where V2 is the secondary-side line voltage (kV), and S rated is the rated capacity of the transformer (MVA).
3. Calculate Transformer Impedance
The transformer impedance ZT is usually given as a percentage and needs to be converted to an actual impedance value. The conversion formula is:
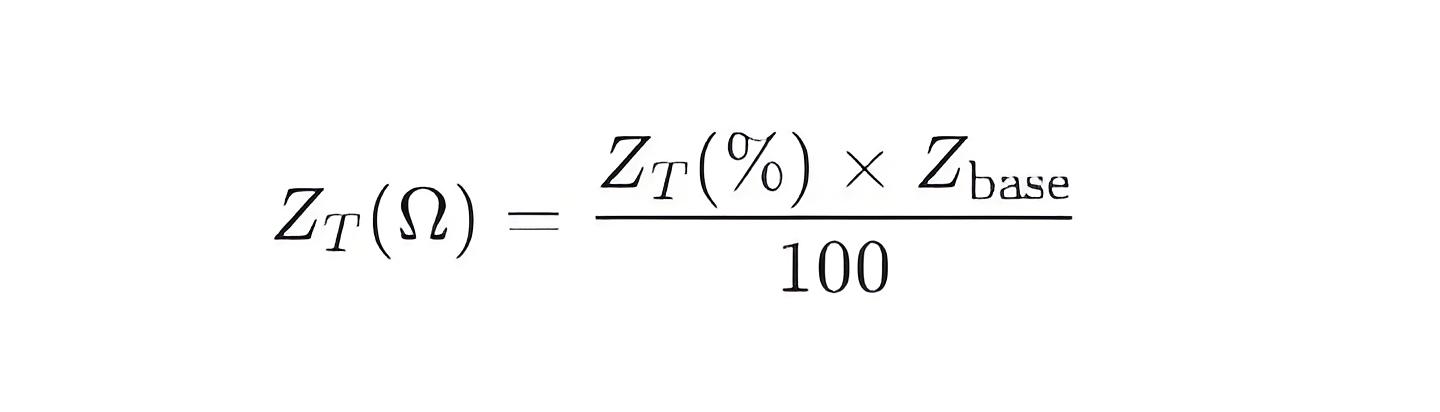
4. Calculate Transmission Line Impedance
If the transmission line impedance is given in ohms per kilometer, calculate the total impedance based on the line length L:
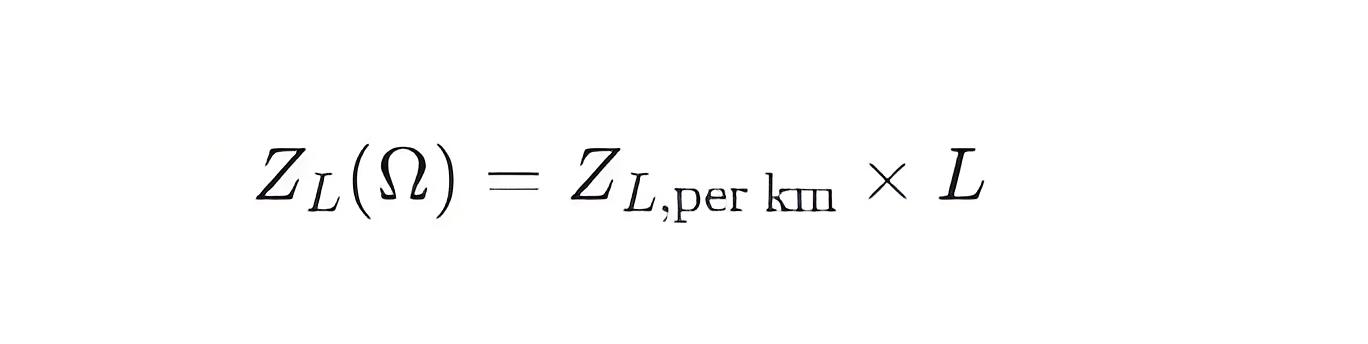
5. Calculate Equivalent Source Impedance
If the equivalent source impedance ZS is known, use it directly. If the source is very strong, you can assume ZS≈0.
6. Calculate Total Impedance
The total impedance Ztotal is the sum of the transformer impedance, transmission line impedance, and equivalent source impedance:

7. Calculate Fault Current
The fault current Ifault can be calculated using Ohm's Law:
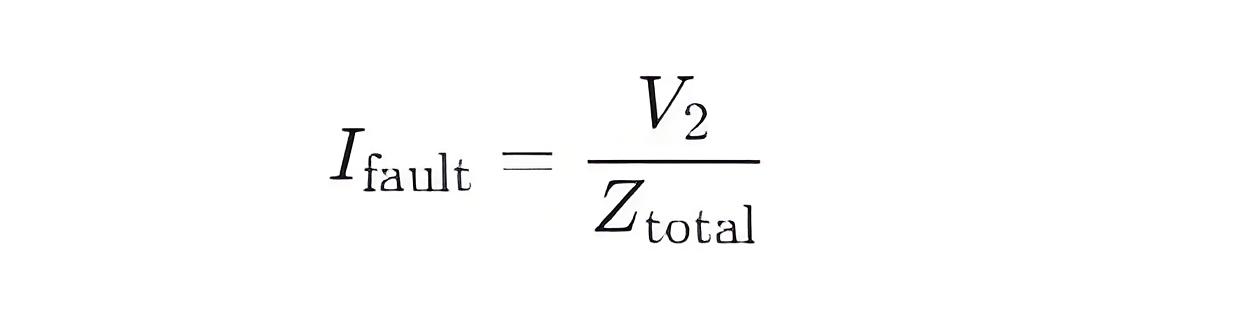
where V2 is the secondary-side line voltage (kV), and Ztotal is the total impedance (ohms).
Note: The calculated I fault is the line current (kA). If you need the phase current, divide by
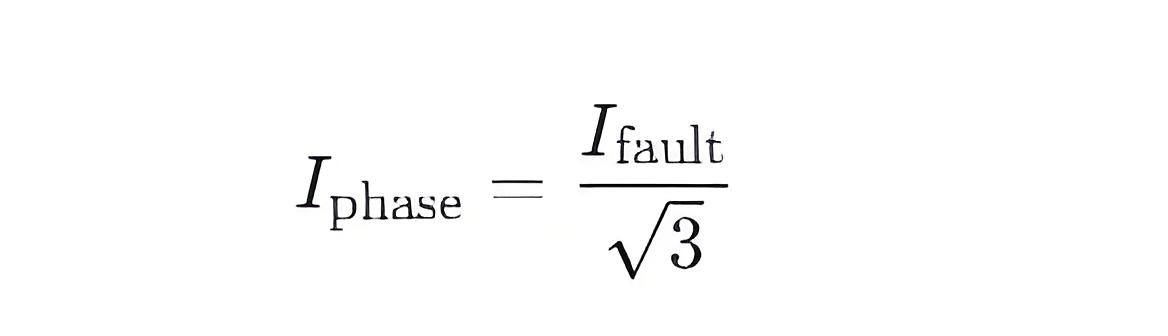
8. Consider System Short-Circuit Capacity
In some cases, it may be necessary to consider the system's short-circuit capacity SC , which can be calculated as:
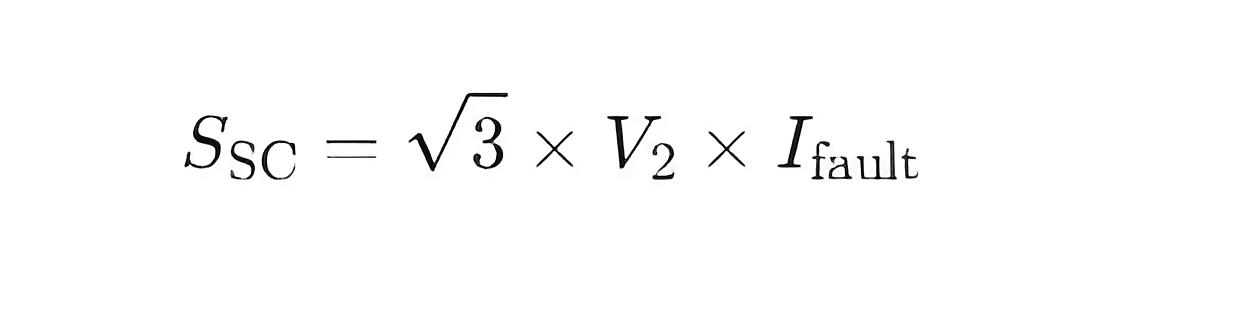
where SC is in MVA.
9. Consider Parallel Transmission Lines
If there are multiple parallel transmission lines, the impedance of each line ZL must be combined in parallel. For n parallel lines, the total transmission line impedance is:
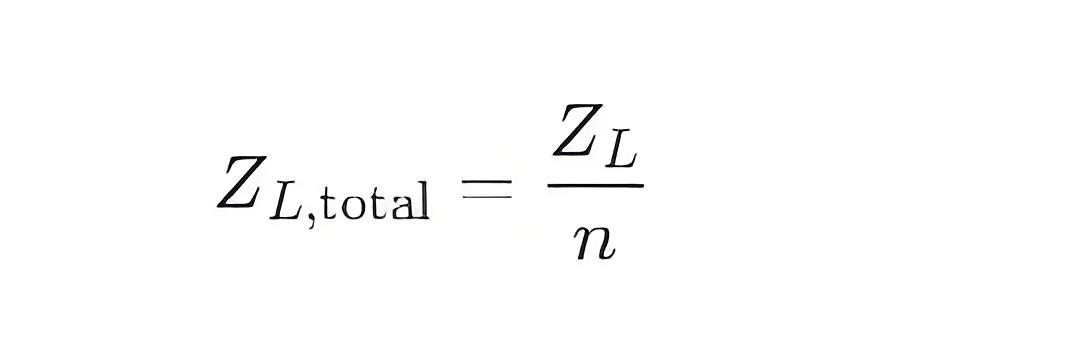
10. Consider Other Factors
Load Impact: In real systems, loads can affect the short-circuit current, but in most cases, load impedance is much larger than the source impedance and can be neglected.
Relay Protection Action Time: The duration of the short-circuit current depends on the action time of relay protection devices, which typically operate within milliseconds to seconds to clear the fault.
Summary
To calculate the fault current on the secondary side of a transformer supplying a transmission line, you need to consider the transformer impedance, transmission line impedance, and equivalent source impedance. By normalizing all impedances to the same base value and applying Ohm's Law, you can calculate the fault current. In practical applications, you should also consider the action time of relay protection devices and the impact of loads.
The Electricity Encyclopedia is dedicated to accelerating the dissemination and application of electricity knowledge and adding impetus to the development and innovation of the electricity industry.